Bách khoa toàn thư mở Wikipedia
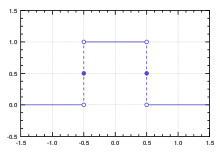 Hàm rect.
Hàm rect.
Hàm chữ nhật hay hàm rect là một hàm toán học liên tục được định nghĩa như sau:[1]
![{\displaystyle \operatorname {rect} (t)=\sqcap (t)={\begin{cases}0&{\text{khi }}|t|>{\frac {1}{2}}\\[3pt]{\frac {1}{2}}&{\mbox{khi }}|t|={\frac {1}{2}}\\[3pt]1&{\text{khi }}|t|<{\frac {1}{2}}.\end{cases}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5b6c1883f64d8b1640bcc507361b7c523678cdb9)
Ngoài ra, trong nhiều lĩnh vực đặc biệt là lĩnh vực xử lý tín hiệu, hàm rect còn được định nghĩa theo cách khác như sau:[2]
![{\displaystyle \operatorname {rect_{d}} (t)={\begin{cases}1&{\text{khi }}|t|\leq {\frac {1}{2}}\\[3pt]0&{\text{khi }}|t|>{\frac {1}{2}}.\end{cases}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/31385cf51ff4238196a62d9d62d0ded24d1dfefb)
Biến đổi Fourier liên tục của hàm rect là một hàm sinc:

và:

Tích chập của 2 hàm rect là 1 hàm tri.

Sử dụng hàm rect như là một hàm mật độ xác suất, nó là 1 trường hợp đặc biệt của phân phối đều liên tục với  .
.
Hàm đặc trưng:

Hàm sinh mômen:

với  là một hàm hypebolic.
là một hàm hypebolic.
Hàm rect có thể được biểu diễn dưới dạng là giới hạn của 1 hàm hữu tỉ:

- Trường hợp
 . Với mọi số nguyên
. Với mọi số nguyên n thì (2t)2n luôn luôn dương. Do 2t<1 cho nên (2t)2n→0 khi n→∝.
- Suy ra:

- Trường hợp
 . Với mọi số nguyên
. Với mọi số nguyên n thì (2t)2n luôn luôn dương. Do 2t>1 cho nên (2t)2n→∝ khi n→∝.
- Suy ra:

- Trường hợp
 .
.
- Dễ dàng ta có:

Từ đó có thể định nghĩa hàm rect như sau:

- ^ Weisstein, Eric W. (ngày 15 tháng 8 năm 2011). “Rectangle Function”. Wolfram MathWorld. Wolfram. Truy cập ngày 15 tháng 8 năm 2011.
- ^ (tiếng Đức)Signalübertragung (ấn bản thứ 6.). Springer Verlag. 1995. tr. 2. ISBN 3-540-54824-6.


![{\displaystyle \operatorname {rect} (t)=\sqcap (t)={\begin{cases}0&{\text{khi }}|t|>{\frac {1}{2}}\\[3pt]{\frac {1}{2}}&{\mbox{khi }}|t|={\frac {1}{2}}\\[3pt]1&{\text{khi }}|t|<{\frac {1}{2}}.\end{cases}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5b6c1883f64d8b1640bcc507361b7c523678cdb9)
![{\displaystyle \operatorname {rect_{d}} (t)={\begin{cases}1&{\text{khi }}|t|\leq {\frac {1}{2}}\\[3pt]0&{\text{khi }}|t|>{\frac {1}{2}}.\end{cases}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/31385cf51ff4238196a62d9d62d0ded24d1dfefb)














